Ryll-Nardzewski fixed-point theorem

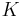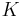In functional analysis, a branch of mathematics, the Ryll-Nardzewski fixed-point theorem states that if E is a normed vector space and K is a nonempty convex subset of E which is compact under the weak topology, then every group (or equivalently: every semigroup) of affine isometries of K has at least one fixed point. (Here, a fixed point of a set of maps is a point that is fixed by each map in the set.)

This theorem was announced by Czesław Ryll-Nardzewski.[1] Later Namioka and Asplund [2] gave a proof based on a different approach. Ryll-Nardzewski himself gave a complete proof in the original spirit.[3]

Applications

The Ryll-Nardzewski theorem yields the existence of a Haar measure on compact groups.[4]

See also

References

  1. ^ Ryll-Nardzewski, C. (1962). "Generalized random ergodic theorems and weakly almost periodic functions". Bull. Acad. Polon. Sci. Sér. Sci. Math. Astronom. Phys. 10: 271–275. 
  2. ^ Namioka, I.; Asplund, E. (1967). "A geometric proof of Ryll-Nardzewski's fixed point theorem". Bull. Amer. Math. Soc. 73 (3): 443–445. doi:10.1090/S0002-9904-1967-11779-8. 
  3. ^ Ryll-Nardzewski, C. (1967). "On fixed points of semi-groups of endomorphisms of linear spaces". Proc. 5-th Berkeley Symp. Probab. Math. Stat (Univ. California Press) 2: 1: 55–61. 
  4. ^ Bourbaki, N. (1981). Espaces vectoriels topologiques. Chapitres 1 à 5. Éléments de mathématique. (New ed.). Paris: Masson. ISBN 2225684103.